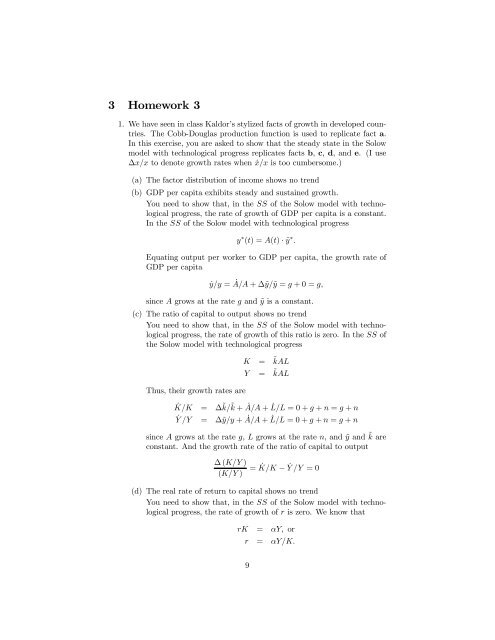
3 Homework 3 - Homepage Usask

3 Homework 3 

1. We have seen in class Kaldor’s stylized facts of growth in developed countries. 

The Cobb-Douglas production function is used to replicate fact a. 

In this exercise, you are asked to show that the steady state in the Solow 

model with technological progress replicates facts b, c, d, ande. (I use 

∆x/x to denote growth rates when ˙x/x is too cumbersome.) 

(a) The factor distribution of income shows no trend 

(b) GDP per capita exhibits steady and sustained growth. 

You need to show that, in the SS of the Solow model with technological 

progress, the rate of growth of GDP per capita is a constant. 

In the SS of the Solow model with technological progress 

y ∗ (t) =A(t) · ˜y ∗ . 

Equating output per worker to GDP per capita, the growth rate of 

GDP per capita 

˙y/y = ˙ A/A + ∆˜y/˜y = g +0=g, 

since A grows at the rate g and ˜y is a constant. 

(c) The ratio of capital to output shows no trend 


progress, the rate of growth of this ratio is zero. In the SS of 

the Solow model with technological progress 

Thus, their growth rates are 

K = ˜ kAL 

Y = ˜ kAL 

˙K/K = ∆ ˜ k/ ˜ k + ˙ 

A/A + ˙ L/L =0+g + n = g + n 

˙Y/Y = ∆˜y/y + ˙ A/A + ˙ L/L =0+g + n = g + n 

since A grows at the rate g, L grows at the rate n, and ˜y and ˜ k are 

constant. And the growth rate of the ratio of capital to output 

∆ (K/Y ) 

(K/Y ) = ˙ K/K − ˙ Y/Y =0 

(d) The real rate of return to capital shows no trend 


progress, the rate of growth of r is zero. We know that 

rK = αY, or 

r = αY/K. 

9

Youjustshowinpartc. that Y/K is a constant and we know that 

α is a constant. Therefore 

˙r ˙α ∆ (K/L) 

= + 

r α (K/L) =0+0=0 

(e) Wages exhibit sustained growth 


progress, the rate of growth of w is a constant. We know 

that 

wL = (1−α)Y, or 

w = (1−α)y. Youjustshowinpartb. that y grows at the rate g and we know 

that (1 − α) is constant. Therefore 

˙w ∆(1 − α) ˙y 

= + =0+g = g. 

w (1 − α) y 

2. (The answer from the textbook is enclosed. A printout for my answer is 

also enclosed: differences between the two answers are due to rounding 

errors) 

It is easier to start calculating the case in which A converges completely; 

i.e., Â =1. The third column in the Table calculates ˆs, the fifth calculates 

h = exp(0.1 ∗ u) andthesixthcalculatesˆ h, the 8th calculates d0 = d + g + 

n =0.075 + n and the 9th calculates ˆ d0. Column 10 then calculates 

µ 1/2 

ˆs 

ˆh 

ˆd0 

using columns 3, 6 and 9. To calculate the case in which the 1990 TFP 

ratios are maintained, we just need to multiply the previous answer by 

the 1990 TFP ratios (columns 10 and 12) to obtain column 13. 

The distance in this case is calculated following your method: 

ˆy ∗ − ˆy97 

ˆy ∗ . 

As you can see the countries are ranked by the rate of growth (or distance) 

in the first case 1 so Cameroon will grow the fastest and the US the slowest. 

In the second case, Argentina is predicted to grow at a rate below "normal" 

(that of the States) and the rank is as follows (from fastest to slowest): 

1) Cameroon, 2) Canada, 3) Thailand, 4) USA, 5) Argentina. (You were 

only asked which economy will be the fastest and which the slowest.) 

1 The answer in the textbook mixes cases a) and b) 

10

3 Empirical Applications of Neoclassical Growth Models 

Exercise 1. Where are these economies headed? 

From equation (3.9), we get 

ˆy ∗ = 

α 

ˆsK 1−α 

ˆh Â = 

ˆx 

ˆsK 

 

(n + 0.075) 

α 

1−α 

e ψ(u−uU.S.) Â, 

where the (ˆ) is used to denote a variable relative to its U.S. value and x = n+g+d. 

The calculations below assume α = 1/3 and ψ = .10, as in the chapter. 

Applying this equation using the data provided in the exercise leads to the 

following results for the two cases: Case (a) maintains the 1990 TFP ratios, while 

case (b) has TFP levels equalized across countries. The Ratio column reports the 

ratio of these steady-state levels to the values in 1997. 

ˆy97 (a) ˆy ∗ Ratio (b) ˆy ∗ Ratio 

U.S.A. 1.000 1.000 1.000 1.000 1.000 

Canada 0.864 1.030 1.193 1.001 1.159 

Argentina 0.453 0.581 1.283 0.300 0.663 

Thailand 0.233 0.554 2.378 0.259 1.112 

Cameroon 0.048 0.273 5.696 0.064 1.334 

The country furthest from its steady state will grow fastest. (Notice that by 

furthest we mean in percentage terms). So in case (a), the countries are ranked by 

their rates of growth, with Cameroon predicted to grow the fastest and the United 

States predicted to grow the slowest. In case (b), Cameroon is still predicted to 

grow the fastest while Argentina is predicted to grow the slowest. 

Exercise 2. Policy reforms and growth. 

The first thing to compute in this problem is the approximate slope of the relationship 

in Figure 3.8. Eyeballing it, it appears that cutting output per worker in 

half relative to its steady-state value raises growth over a 37-year period by about 2 

percentage points. (Korea is about 6 percent growth, countries at the 1/2 level are 

about 4 percent, and countries in their steady state are about 2 percent). 

10

y97 s s-hat u h h-hat n d' d'-hat y* (A-hat=1) distance A90 y* (A-hat90) distance 

USA 1 0.204 1.000 11.9 3.287 1.000 0.01 0.085 1.000 1.000 0.000 1 1.000 0.000 

Canada 0.864 0.246 1.206 11.4 3.127 0.951 0.012 0.087 1.024 1.033 0.163 0.972 1.004 0.139 

Argentina 0.453 0.144 0.706 8.5 2.340 0.712 0.014 0.089 1.047 0.584 0.225 0.517 0.302 -0.499 

Thailand 0.233 0.213 1.044 6.1 1.840 0.560 0.015 0.09 1.059 0.556 0.581 0.468 0.260 0.105 

Cameroon 0.048 0.102 0.500 3.4 1.405 0.427 0.028 0.103 1.212 0.275 0.825 0.234 0.064 0.253

3. Unemployment and growth. Consider how unemployment would affect 

the Solow growth model. Suppose that output is produced according 

to the production function Y = K α [(1−u ∗ )L] 1−α ,whereu ∗ is the natural 

rate of unemployment. There is no technological progress. Assume again 

that the labour force equals population. 

(a) Express output per worker, y, as a function of capital per worker, k, 

and the natural rate of unemployment. Describe the steady state of 

this economy. 

Output per worker equals 

y = Y 

L = Kα (1 − u∗ ) 1−αL1−α =(1−u 

L 

∗ ) 1−α 

µ α 

K 

=(1−u 

L 

∗ ) 1−α k α . 

A decrease in the natural rate of unemployment increases output 

per worker y at all levels of capital per worker k since there is more 

output to divide about: the production function curves shifts up. 

(Remember that workers are everybody in the labour force, not only 

the employed ones.) 

Since output per worker increases at all levels of k, investmentincreases 

at all levels of k, other things being equal (the investment 

curves shifts up); therefore, the capital per worker in the steady state, 

k ∗ , depends on the natural rate of unemployment. 

(Analytically, the steady state condition is the same: 

substituting 

sy = d 0 k; 

s(1 − u ∗ ) 1−α k α = d 0 k 

and solving for k 

k ∗ ³ 

s 

= 

d0 You were not required to do this part.) 

´ 1 

1−α 

(1 − u ∗ ). 

(b) Suppose that some change in government policy reduces the natural 

rate of unemployment. Describe how this change affects output both 

immediately and over time. Is the steady-state effect on output larger 

or smaller than the immediate effect? Explain (Your answer should 

include a graph). 

The immediate effect is the increase in output per worker y at the 

same level of capital per capita k ∗ 1 (the old steady state capital per 

capita), from y∗ 1 to y0 2 =(1−u∗ 2) 1−αk∗ 1. However, this immediate 

effect also increases savings from s · y∗ 1 to s · y0 2 >d0 · k∗ 1 , greater than 

depreciation; therefore, there exists net investment which will start 

the process of accumulating more capital and moving to a higher 

steady state; i.e., the eventual effect (steady state) includes the extra 

increase in output due to the consequent increase in capital. 

11

(Analytically, suppose that the natural rate of unemployment is u ∗ 1.In 

the steady state, 

´ 1 

1−α 

k ∗ 1 = 

³ 

s 

d0 y ∗ 1 = 

³ 

s 

d0 The first effect would be 

y = (1−u ∗ 2) 1−α 

∙ ³ 

s 

d0 (1 − u ∗ 2) 1−α ³ s 

d0 (1 − u ∗ 1) and 

´ α 

1−α 

(1 − u ∗ ). 

but eventually output per capita equals 

y ∗ 2 = 

´ 1 

1−α 

(1 − u ∗ ¸α 

1) = 

´ α 

1−α 

(1 − u ∗ 1) α 

³ 

s 

d0 ´ 1 

1−α 

(1 − u ∗ 2). 

Again you were not required to do this.) 

4. The answer is enclosed. 

12

From the Mankiw-Romer-Weil (1992) model, we have the production function: 

Divide both sides by AL to get 

Y = K α H β (AL) 1−α−β . 

y 

A = 

α β k h 

. 

A A 

Using the (˜) to denote the ratio of a variable to A, this equation can be rewritten as 

˜y = ˜ k α˜ h β . 

Now turn to the capital accumulation equation: 

˙K = sKY − dK. 

As usual, this equation can be written to describe the evolution of ˜ k as 

˙˜k = sK ˜y − (n + g + d) ˜ k. 

Similarly, we can obtain an equation describing the evolution of ˜ h as 

and 

˙˜h = sH ˜y − (n + g + d) ˜ h. 

In steady state, ˙˜ k = 0 and ˙˜ h = 0. Therefore, 

˜k = 

˜h = 

sK 

n + g + d ˜y, 

sH 

n + g + d ˜y. 

Substituting this relationship back into the production function, 

˜y = ˜ k α˜ 

 

β sK 

h = 

n + g + d ˜y 

α 

sH 

n + g + d ˜y 

Solving this equation for ˜y yields the steady-state level 

˜y ∗ α 

sK 

sH 

= 

n + g + d n + g + d 

β 

. 

β 1 

1−α−β 

. 

14

Finally, we can write the equation in terms of output per worker as 

y ∗ α 

sK 

sH 

(t) = 

n + g + d n + g + d 

Compare this expression with equation (3.8), 

y ∗ 

sK 

(t) = 

n + g + d 

α 

1−α 

hA(t). 

β 1 

1−α−β 

A(t). 

In the special case β = 0, the solution of the Mankiw-Weil-Romer model is 

different from equation (3.8) only by a constant h. Notice the symmetry in the 

model between human capital and physical capital. In this model, human capital 

is accumulated by foregoing consumption, just like physical capital. In the model 

in the chapter, human capital is accumulated in a different fashion — by spending 

time instead of output. 

15

3 Homework 3 - Homepage Usask

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?